Limit of a Function
The limit of a function at a point a a a in its domain if it exists is the value that the function approaches as its argument approaches a. Consider the function for values of near 2.
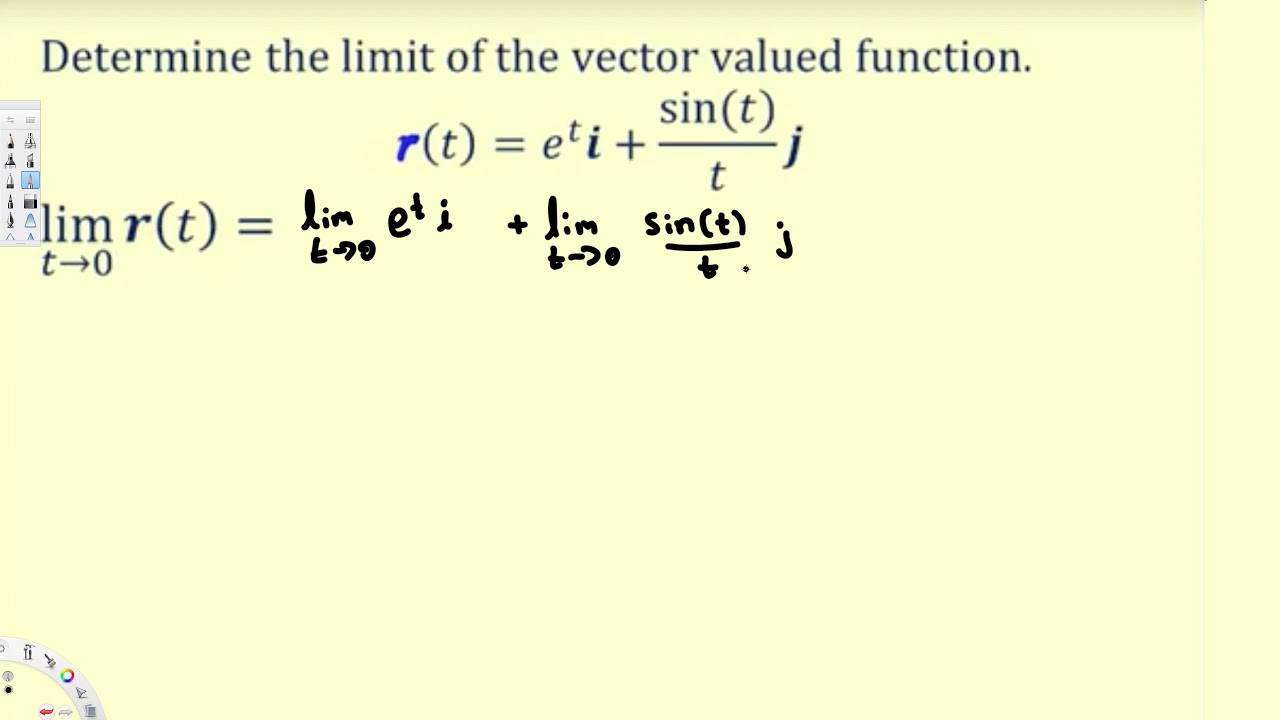
Limit Of Vector Valued Functions 1 Vector Calculus Calculus Vector
HeyThis video is all about Indeterminate Forms And L Hospitals Rulepart 1 Indeterminate Forms And L Hospitals Rulehttpsyoutube0UGp11RedRgLink Fo.

. The limit does not exist at a We cant say what the value at a is because there are two competing answers. Definition 133 Informal definition of limit. A one-sided limit is a value the function approaches as the x-values approach the limit from one side only.
The limit is. If it sounds complicated its actually a cake walk compared to trying to use algebra to find limit of sums. In the case where x approaches 2 how does this.
If the value of the function fx f x is sure to be arbitrarily close to L L whenever the value of x x is close. 223 Use a graph to estimate the limit of a function or to identify when the limit. The right limit of a function ƒx is A as x approaches a from the right lim x a ƒx A Left Limit of a Function Notation.
Limit of a function example of Trigonometric Functions. Advanced Math Solutions Limits Calculator Factoring In a previous post we talked about using substitution to find the limit of a function. Limits are important in calculus and mathematical analysis and used to define.
If your limit is multiply the numerator and denominator with to get. Use and separate the multiplied fractions to obtain. Solving Limit of a Function.
The second part of the fundamental theorem of calculus gives you the indefinite integral. 38 from the left and. When the value of a variable x Is brought closer and closer to a number a the value of function f x approaches closer and closer to a definite number l.
How about a function fx with a break in it like this. The calculator will use the best method available so try out a lot of different types of problems. This section introduces the formal definition of a limit.
The common phrase the. Finding the limit by Factorization. The limit of a function at is.
The limit notation for the two problems from the last section is lim x1 22x2 x 1 4 lim t5 t36t225 t 5 15 lim x 1 2 2 x 2 x 1 4 lim t 5 t 3 6 t 2 25 t. The Limit Calculator supports find a limit as x approaches any number including infinity. You can plug in to get.
Finding limit by plugging the value of x. Many refer to this as the epsilon-delta definition referring to the letters ε and δ of the Greek alphabet. The limit of a function is a fundamental concept in calculus and analysis concerning the behavior of the function near a particular value of its independent variable.
Lim xafx L lim x a f x L. The concept of a limit is the fundamental concept of calculus and analysis. In Mathematics a limit is defined as a value that a function approaches the output for the given input values.
Supposing m is a real number in the. For example f xxx returns -1 for negative numbers 1 for positive. 222 Use a table of values to estimate the limit of a function or to identify when the limit does not exist.
Limit of a function. The left limit of a function ƒx is A as x approaches a from the. There are barely any significant cutoff properties that are associated with geometrical capacities.
Finding Limit by rationalization. This function is a parabola which we know because it contains a variable to the second power.
Limits And Continuity For Multivariable Functions Vector Calculus Vector Calculus Calculus Differential Calculus

Calculus Limits At Infinity Rational Functions Four Easy Examples Rational Function Calculus Math Videos

Limit Of An Irrational Function With A Square Root Term Square Roots Term Math

Formal And Epsilon Delta Definition Of Limit Of A Function With Examples Maths Algebra Formulas Math Books Physics And Mathematics

Limits Of Vector Valued Functions Vector Calculus 2

Limit Of Vector Valued Functions Vector Calculus 3 Vector Calculus Calculus School Help
0 Response to "Limit of a Function"
Post a Comment